Multiplication of Decimal Numbers

On this Page:
1) Power of 10 Multiplication
2) Multiplying Decimals Together
3) Examples
4) Decimal Long Multiplication

Multiplication of decimal numbers can often be something that looks potentially tough at first, but really turns out to be more straight forward than initially feared.

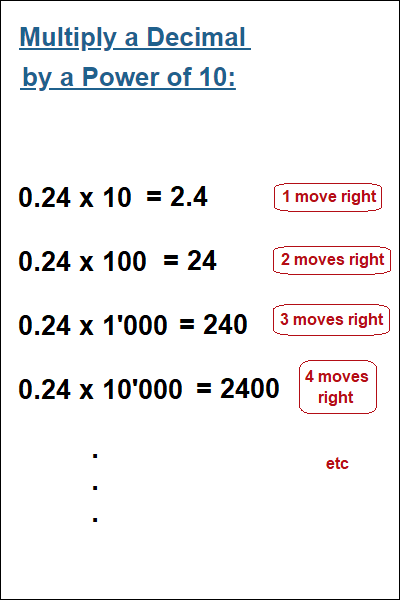
Power of 10 Decimal Multiplication

We can consider the following multiplication sum, 0.24 × 100 = ?

This is a fairly basic multiplication of decimal numbers sum, with multiplication by a power of 10.

The number 100 contains 2 zeroes, for us this means that the decimal point will be moving 2 places to the right in 0.24.

0.24 × 100 => 1) 2.4 => 2) 24.

0.24 × 100 = 24

Now 24.0 is just 24 on its own.

In the same way, if 0.24 was multiplied by 1000:

0.24 × 1000 = 240

Summary

Decimals Multiplied Together

We can look at the following. 0.003 × 0.9 = ?

An effective method is to initially multiply the numbers that are in the sum, but with no decimal points present.

3 × 9 = 27

Then count how many digits there are after the decimal point in both of the original decimal numbers in the multiplication sum combined.

0.003 × 0.9
^{1 2 3} ⁴

There are 4 digits after the decimal point in both numbers together from the original multiplication sum.

So we will place a decimal point to the right of the 27 we worked out, and then move this decimal point 4 places to the left.

27. => 2.7 ( 1 move ) => 0.27 ( 2 moves ) => 0.027 ( 3 moves ) => 0.0027 ( 4 moves )

0.003 × 0.9 = 0.0027

Multiplication of Decimal Numbers Examples

1.1

0.5 × 5

Solution

5 × 5 = 25
0.5 × 5
¹

25. => 2.5 ( 1 move )
0.5 × 5 = 2.5

1.2

0.2 × 80

Solution

2 × 80 = 120
0.2 × 80
¹

120. => 12.0 ( 1 move )
0.2 × 80 = 12

1.3

0.4 × 3.2

Solution

4 × 32 = 128
0.4 × 3.2
¹ ²

128. => 12.8 ( 1 move ) => 1.28 ( 2 moves )
0.4 × 3.2 = 1.28

1.4

0.04 × 0.006

Solution

4 × 6 = 24
0.04 × 0.006
¹ ² ³ ⁴ ⁵

24. => 2.4 ( 1 move ) => 0.24 ( 2 moves ) => 0.024 ( 3 moves )
=> 0.0024 ( 4 moves ) => 0.00024 ( 5 moves )

0.04 × 0.006 = 0.00024

Long Multiplication with Decimals

2.1

14.5 × 2.1 = ?

For situations where multiplying decimals involves long multiplication.
The same process as using whole non decimal numbers also applies.
We multiply as if using regular whole numbers, then move the decimal point to the correct position in the answer.

Solution

(step 1) (step 2)

\begin{array}{r} \space{\bf{145}}\space\space\\ \times\space\space\space\space\space\space{\bf{1}}\space\space\\ \hline {\bf{145}}\space\space \end{array}

\begin{array}{r} \space{\bf{145}}\space\space\\ \times\space\space\space\space\space\space{\bf{2}}\space\space\space\space\\ \hline {\bf{2900}}\space\space \end{array}

(step 3)

Adding both results:

\begin{array}{r} {\bf{145}}\space\space\\ +\space\space\space\space\space{\bf{2900}}\space\space\\ \hline {\bf{3045}}\space\space \end{array}

14.5 × 2.1
¹ ²
We move the decimal point 2 places to the left.

3045. => 30.45

14.5 × 2.1 = 30.45

Home
Fractions and Decimals

Return to TOP of page