When dealing with certain sums, it’s often important to know how to perform the correct arithmetic order of operations.
For example, with the following sum. 4^2 \space{\text{--}}\space 5 \times 3
In what order should you perform the power, minus, and multiplication?
We could possibly proceed as follows.
3^2 \space{\text{--}}\space 2 \times 4 \space = \space 16 \space{\text{--}}\space 5 \times 3 \space = \space 11 \times 3 \space = \space 33
Or
3^2 \space{\text{--}}\space 2 \times 4 \space = \space 16 \space{\text{--}}\space 5 \times 3 \space = \space 16 \space {\text{--}} \space 15 \space = \space 1
But which is correct?
We need to be sure that you are doing each operation at the right stage in the correct order, getting the Order of Operations right.
The operations in most standard sums are addition and subtraction, multiplication and division, along with powers/exponents. Sometimes the presence of brackets can also affect a sum too.
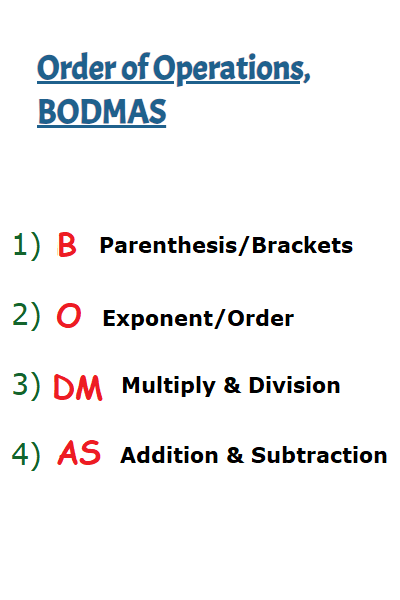
Order of Operations BODMAS
The word ‘BODMAS‘ can be a quick and easy way to correctly remember the right order of operations.
The word is in fact an abbreviation, where each letter represents an operation in a sum.
Each letter present, occurs in the order of when to do the operation, from first to last.
1) B Brackets / Parenthesis
2) O Order / Exponent
3) DM Multiplication AND Division
4) AS Addition AND Subtraction
Now as can be seen, Multiplication and Division are ranked together, and so are Addition and Subtraction.
When these operations feature in the same sum. The order in which to perform each operation is just from left to right, as they occur in the sum.
So 24 \div 4 \times 2 is NOT 24 \div ( 4 \times 2 ) = 24 \div 8 = 3
But is instead the following.
24 \div 4 \times 2 = ( 24 \div 4 ) \times 2 \space = \space 6 \times 2 \space = \space 12
Order of Operations BODMAS
Examples
1.1
3^2 \space {\text{--}} \space 4
2^2 first, then minus 4.
2^2 \space {\text{--}} \space 1 = 4 \space {\text{--}} \space 1 = 3
1.2
(1+2)^3 + 5
Deal with what’s inside the parenthesis/brackets first.
Then the power, then plus 2.
(1+2)^3 + 5 \space = \space 3^3 + 5 \space = \space 9 + 5 \space = \space 14
1.3
(5+1)^2 \div 2
Division does out rank addition, but the addition is in the brackets, so is dealt with first.
Then the power, then division by 2.
(5+1)^2 \div 2 \space = \space 6^2 \div 2 \space = \space 36 \div 2 \space = \space 18
1.4
2 \times 3^2 + 1
3^2 is dealt with first, then the multiplication by 2, then plus 1.
2 \times 3^2 + 1 \space = \space 2 \times 9 + 1 \space = \space 18 + 1 \space = \space 19
1.5
4 + 7^2 \times 2 \space = \space 4 + 49 \times 2 \space = \space 4 + 98 \space = \space 102
1.6
5 \space {\text{--}} \space 1 + 4
With order of operations BODMAS, both operations have the same rank, thus are carried out left to right.
5 \space {\text{--}} \space 1 + 4 \space = \space 4 + 4 \space = \space 8
Summary